Aristotle’s Wheel Paradox: When Geometry Challenges Common Sense
Published on May 2, 2025
Aristotle’s Wheel Paradox is one of the most thought-provoking puzzles in ancient philosophy and geometry. At first glance, it looks like a simple observation of rolling wheels — but upon closer inspection, it reveals a contradiction that has puzzled thinkers for centuries.
What Is the Paradox?
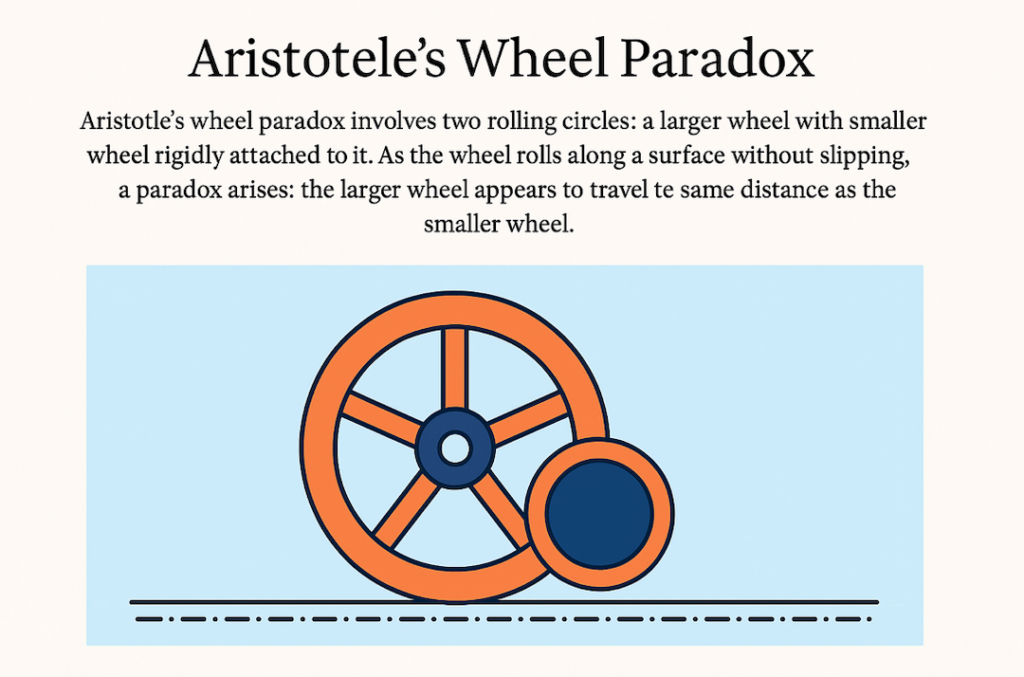
Imagine two wheels, one larger and one smaller, rigidly attached to each other so that they rotate together without slipping. As the combined wheel rolls along a flat surface, logic suggests that the larger wheel, having a bigger circumference, should travel a greater distance than the smaller one.
However, when observed in motion, both wheels appear to trace the same linear distance on the ground — even though their circumferences are clearly different.
Why Is This a Paradox?
The core of the paradox lies in the relationship between rotation and distance. If the smaller wheel rotates once, and the larger wheel (connected to it) also rotates once, they both should have traveled their respective circumferences.
But how can they roll side-by-side and cover the same distance? The answer depends on how you measure “motion” and whether you allow for slipping or not.
What Does It Teach Us?
This paradox is not just a curiosity — it challenges how we interpret physical space, ideal motion, and even measurement itself. Mathematicians often use it to teach the concept of isometry, non-slip conditions, and the subtleties of circular motion.
Visual Representation
The illustration above shows how both wheels roll simultaneously — yet their contact points suggest equal distances covered.
Final Thought
Aristotle’s Wheel Paradox reminds us that intuition and geometry can sometimes collide. In the world of perfect forms and thought experiments, not everything behaves the way we might expect.

